Ohm’s Law:
Ohm’s Law states that Electromotive Force (Voltage) is equal to Current multiplied by Resistance.
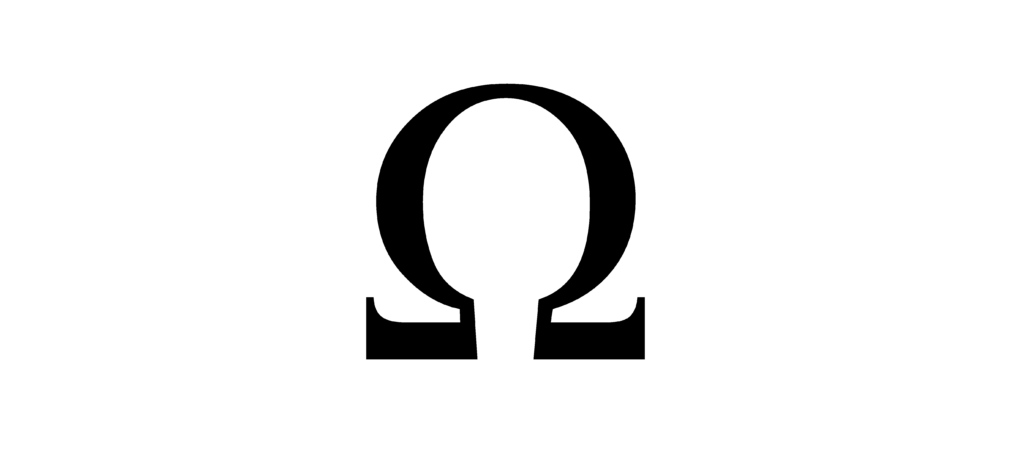
$$E_{volts(V)}=I_{amps(A)}\times R_{ohms(\Omega)}$$
Simplified:
$$E=I\times R$$
We can now manipulate the above equation to solve for Current.
$$I_{amps(A)}=\frac{E_{volts(V)}}{R_{ohms(\Omega)}}$$
Simplified:
$$I=\frac{E}{R}$$
And also Resistance.
$$R_{ohms(\Omega)}=\frac{E_{volts(V)}}{I_{amps(A)}}$$
Simplified:
$$R=\frac{E}{I}$$
Power Formulas:
The power formula states that Power is equal to Electromotive Force multiplied by Current.
$$P_{watts(W)}=I_{amps(A)}\times E_{volts(V)}$$
Simplified:
$$P=I\times E$$
We can now manipulate the above equation to solve for Voltage.
$$E_{volts(V)}=\frac{P_{watts(W)}}{I_{amps(A)}}$$
Simplified:
$$E=\frac{P}{I}$$
And also Current.
$${I_{amps(A)}}=\frac{P_{watts(W)}}{E_{volts(V)}}$$
Simplified:
$${I}=\frac{P}{E}$$
Combining Ohm’s Law and Power Formulas to derive new and useful Power formulas:
Power in terms of Voltage and Resistance:
$$P_{watts(W)}=E_{volts(V)}\times I_{amps(A)}$$
$${I_{amps(A)}}=\frac{E_{volts(V)}}{R_{ohms(\Omega)}}$$
Now substitute the I formula into the P formula.
$$P_{watts(W)}=E_{volts(V)}\times \frac{E_{volts(V)}}{R_{ohms(\Omega)}}$$
$$P_{watts(W)}=\frac{E_{volts(V)}^2}{R_{ohms(\Omega)}}$$
Simplified:
$${P=\frac{V^2}{R}}$$
Power in terms of Current and Resistance:
$$P_{watts(W)}=E_{volts(V)}\times I_{amps(A)}$$
$$E_{volts(V)}=I_{amps(A)}\times R_{ohms(\Omega)}$$
Now substitute the E formula into the P formula.
$$P_{watts(W)}=I_{amps(A)}\times R_{ohms(\Omega)}\times I_{amps(A)}$$
$$P_{watts(W)}=I_{amps(A)}^2\times R_{ohms(\Omega)}$$
Simplified:
$${P=I^2 \times R}$$
Impressive!